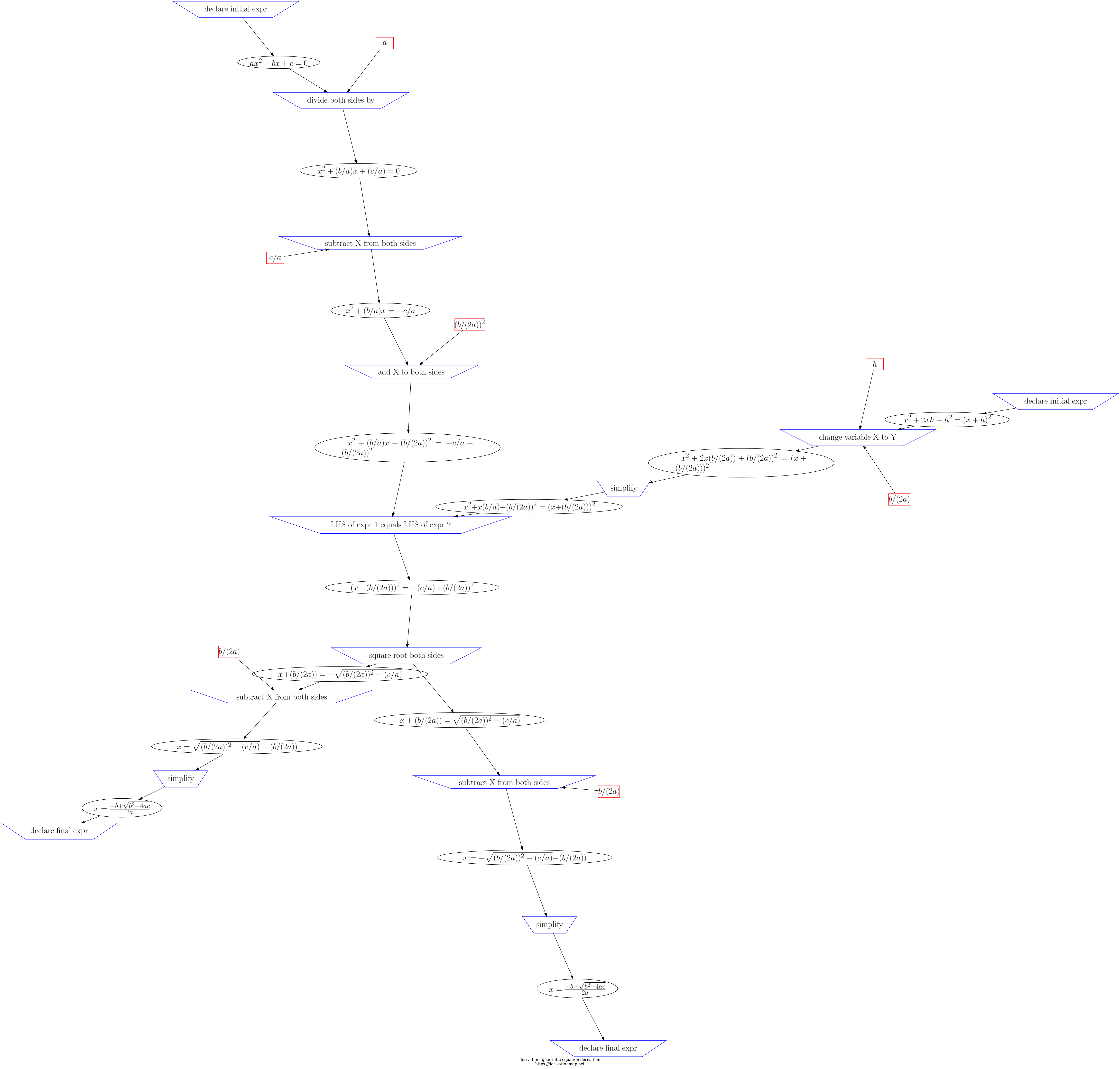
This page contains three views of the steps in the derivation: d3js, graphviz PNG, and a table.
Notes for this derivation:
https://en.wikipedia.org/wiki/Quadratic_formula#Derivations_of_the_formula
| Index | Inference Rule | Input latex | Feeds latex | Output latex | step validity | dimension check | unit check | notes |
|---|---|---|---|---|---|---|---|---|
| 10 | subtract X from both sides |
|
|
|
LHS diff is 0 RHS diff is -sqrt((pdg1939**2 - 4*pdg4231*pdg9139)/pdg9139**2) |
5982958249:
9582958293: |
5982958249:
9582958293: |
|
| 10.5 | subtract X from both sides |
|
|
|
LHS diff is 0 RHS diff is sqrt((pdg1939**2 - 4*pdg4231*pdg9139)/pdg9139**2) |
9582958294:
5982958248: |
9582958294:
5982958248: |
|
| 11 | simplify |
|
|
|
LHS diff is 0 RHS diff is (-pdg9139*sqrt((pdg1939**2 - 4*pdg4231*pdg9139)/pdg9139**2) + sqrt(pdg1939**2 - 4*pdg4231*pdg9139))/(2*pdg9139) |
5982958248:
9999999968: |
5982958248:
9999999968: |
|
| 11.5 | simplify |
|
|
|
LHS diff is 0 RHS diff is (pdg9139*sqrt((pdg1939**2 - 4*pdg4231*pdg9139)/pdg9139**2) - sqrt(pdg1939**2 - 4*pdg4231*pdg9139))/(2*pdg9139) |
9582958293:
9999999969: |
9582958293:
9999999969: |
|
| 5 | add X to both sides |
|
|
|
valid |
5938459282:
5928292841: |
5938459282:
5928292841: |
|
| 4 | subtract X from both sides |
|
|
|
LHS diff is pdg1464*(-pdg1939 + pdg9139)/pdg9139 RHS diff is 0 |
5958392859:
5938459282: |
5958392859:
5938459282: |
|
| 9 | square root both sides |
|
|
|
no check performed |
9385938295:
5982958249: 9582958294: |
9385938295:
5982958249: 9582958294: |
|
| 8 | LHS of expr 1 equals LHS of expr 2 |
|
|
|
input diff is 0 diff is (pdg1464**2*pdg9139 + pdg1464*pdg1939 + pdg4231)/pdg9139 diff is -(pdg1464**2*pdg9139 + pdg1464*pdg1939 + pdg4231)/pdg9139 |
5928292841:
5959282914: 9385938295: |
5928292841:
5959282914: 9385938295: |
|
| 7 | simplify |
|
|
|
valid |
5928285821:
5959282914: |
5928285821:
5959282914: |
|
| 6 | change variable X to Y |
|
|
|
valid |
8582954722:
dimensions are consistent 5928285821: |
8582954722:
N/A 5928285821: |
|
| 14 | declare final expr |
|
|
|
no validation is available for declarations |
9999999968:
|
9999999968:
|
|
| 15 | declare final expr |
|
|
|
no validation is available for declarations |
9999999969:
|
9999999969:
|
|
| 3 | divide both sides by |
|
|
|
LHS diff is pdg1464*(pdg1939 - pdg9139)/pdg9139 RHS diff is 0 |
9285928292:
5958392859: |
9285928292:
5958392859: |
|
| 1 | declare initial expr |
|
|
|
no validation is available for declarations |
9285928292:
|
9285928292:
|
|
| 7.5 | declare initial expr |
|
|
|
no validation is available for declarations |
8582954722:
dimensions are consistent |
8582954722:
N/A |
| symbol ID | category | latex | scope | dimension | name | value | Used in derivations | references | |
|---|---|---|---|---|---|---|---|---|---|
| 9139 | variable |
a
\(a\) |
['real'] | dimensionless | 45 | ||||
| 3410 | variable |
h
\(h\) |
real | dimensionless | none |
|
2 | ||
| 4231 | variable |
c
\(c\) |
['real'] | dimensionless | 12 | ||||
| 1464 | variable |
x
\(x\) |
['real'] | dimensionless |
|
140 | |||
| 1939 | variable |
b
\(b\) |
['real'] | dimensionless | 20 |