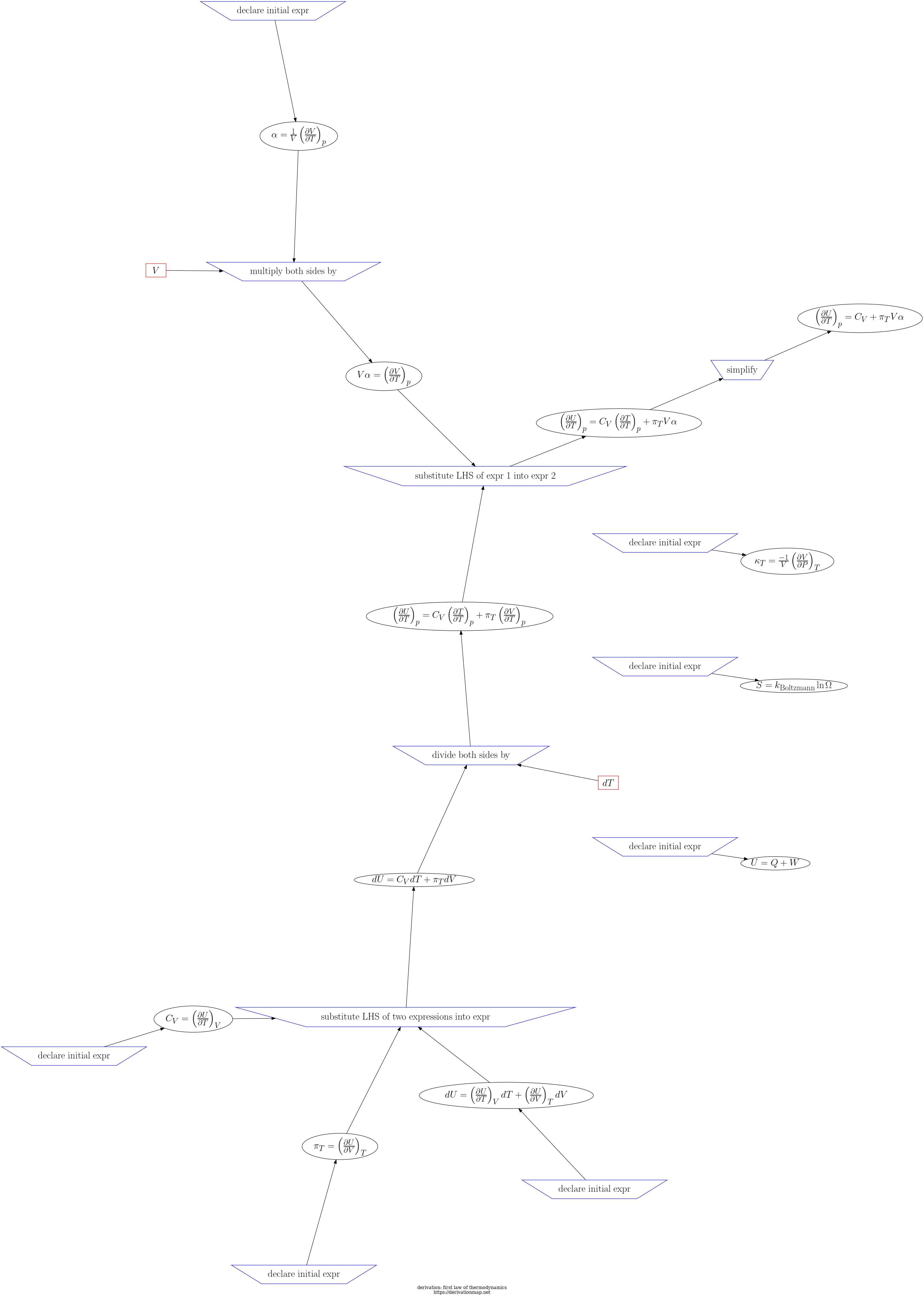
Physics Derivation Graph navigation Sign in

review derivation: first law of thermodynamics

This page contains three views of the steps in the derivation: d3js, graphviz PNG, and a table.


Hold the mouse over a node to highlight that node and its neighbors. You can zoom in/out. You can pan the image. You can move nodes by clicking and dragging.

Notes for this derivation:
https://www.youtube.com/watch?v=QTiqF-HtkS0 and https://www.youtube.com/watch?v=3Yls-t3B49U

Options
Alternate views of this derivation:
Edit this content:    

To edit a step, click on the number in the "Index" column in the table below

Clicking on the step index will take you to the page where you can edit that step.

Index Inference Rule Input latex Feeds latex Output latex step validity dimension check unit check notes
9 multiply both sides by
  1. 3464107376; locally 2714175:
    \(\alpha = \frac{1}{V} \left( \frac{\partial V}{\partial T} \right)_p\)
    \(pdg_{4686} = \frac{\frac{d}{d pdg_{7343}} pdg_{7586}}{pdg_{7586}}\)
  1. 5074423401:
    \(V\)
    \(pdg_{7586}\)
  1. 6397683463; locally 7939101:
    \(V \alpha = \left( \frac{\partial V}{\partial T} \right)_p\)
    \(pdg_{4686} pdg_{7586} = \frac{d}{d pdg_{7343}} pdg_{7586}\)
valid 3464107376:
6397683463:
3464107376:
6397683463:
6 substitute LHS of two expressions into expr
  1. 1085150613; locally 4576755:
    \(C_V = \left(\frac{\partial U}{\partial T}\right)_V\)
    \(pdg_{6682} = \frac{d}{d pdg_{7343}} pdg_{5786}\)
  2. 5634116660; locally 7384950:
    \(\pi_T = \left(\frac{\partial U}{\partial V}\right)_T\)
    \(pdg_{5480} = \frac{d}{d pdg_{7586}} pdg_{5786}\)
  3. 9941599459; locally 2445123:
    \(dU = \left(\frac{\partial U}{\partial T}\right)_V dT + \left(\frac{\partial U}{\partial V}\right)_T dV\)
    \(dU = \frac{d}{d pdg_{7343}} pdg_{5786}\)
  1. 5002539602; locally 5358683:
    \(dU = C_V dT + \pi_T dV\)
    \(dU = dT pdg_{6682} + dV pdg_{5480}\)
failed 1085150613:
5634116660:
9941599459:
5002539602:
1085150613:
5634116660:
9941599459:
5002539602:
8 declare initial expr
  1. 3464107376; locally 2714175:
    \(\alpha = \frac{1}{V} \left( \frac{\partial V}{\partial T} \right)_p\)
    \(pdg_{4686} = \frac{\frac{d}{d pdg_{7343}} pdg_{7586}}{pdg_{7586}}\)
no validation is available for declarations 3464107376:
3464107376:
1 declare initial expr
  1. 1815398659; locally 7368252:
    \(U = Q + W\)
    \(pdg_{5786} = pdg_{1088} + pdg_{9432}\)
no validation is available for declarations 1815398659:
1815398659:
7 divide both sides by
  1. 5002539602; locally 5358683:
    \(dU = C_V dT + \pi_T dV\)
    \(dU = dT pdg_{6682} + dV pdg_{5480}\)
  1. 8854422847:
    \(dT\)
    \(pdg_{7343}\)
  1. 6055078815; locally 3830663:
    \(\left(\frac{\partial U}{\partial T}\right)_p = C_V \left(\frac{\partial T}{\partial T}\right)_p + \pi_T \left( \frac{\partial V}{\partial T} \right)_p\)
    \(\frac{d}{d pdg_{7343}} pdg_{5786}\)
Nothing to split 5002539602:
6055078815:
5002539602:
6055078815:
11 simplify
  1. 2257410739; locally 1136968:
    \(\left(\frac{\partial U}{\partial T}\right)_p = C_V \left(\frac{\partial T}{\partial T}\right)_p + \pi_T V \alpha\)
    \(\frac{d}{d pdg_{7343}} pdg_{5786}\)
  1. 7826132469; locally 1189259:
    \(\left(\frac{\partial U}{\partial T}\right)_p = C_V + \pi_T V \alpha\)
    \(\frac{d}{d pdg_{7343}} pdg_{5786}\)
Nothing to split 2257410739:
7826132469:
2257410739:
7826132469:
5 declare initial expr
  1. 5634116660; locally 7384950:
    \(\pi_T = \left(\frac{\partial U}{\partial V}\right)_T\)
    \(pdg_{5480} = \frac{d}{d pdg_{7586}} pdg_{5786}\)
no validation is available for declarations 5634116660:
5634116660:
10 substitute LHS of expr 1 into expr 2
  1. 6397683463; locally 7939101:
    \(V \alpha = \left( \frac{\partial V}{\partial T} \right)_p\)
    \(pdg_{4686} pdg_{7586} = \frac{d}{d pdg_{7343}} pdg_{7586}\)
  2. 6055078815; locally 3830663:
    \(\left(\frac{\partial U}{\partial T}\right)_p = C_V \left(\frac{\partial T}{\partial T}\right)_p + \pi_T \left( \frac{\partial V}{\partial T} \right)_p\)
    \(\frac{d}{d pdg_{7343}} pdg_{5786}\)
  1. 2257410739; locally 1136968:
    \(\left(\frac{\partial U}{\partial T}\right)_p = C_V \left(\frac{\partial T}{\partial T}\right)_p + \pi_T V \alpha\)
    \(\frac{d}{d pdg_{7343}} pdg_{5786}\)
Nothing to split 6397683463:
6055078815:
2257410739:
6397683463:
6055078815:
2257410739:
2 declare initial expr
  1. 9941599459; locally 2445123:
    \(dU = \left(\frac{\partial U}{\partial T}\right)_V dT + \left(\frac{\partial U}{\partial V}\right)_T dV\)
    \(dU = \frac{d}{d pdg_{7343}} pdg_{5786}\)
no validation is available for declarations 9941599459:
9941599459:
hold volume constant in first term; hold temperature constant in second term
3 declare initial expr
  1. 3547519267; locally 8155541:
    \(S = k_{\rm Boltzmann} \ln \Omega\)
    \(pdg_{1394} = pdg_{1157} \log{\left(pdg_{3434} \right)}\)
no validation is available for declarations 3547519267:
3547519267:
4 declare initial expr
  1. 1085150613; locally 4576755:
    \(C_V = \left(\frac{\partial U}{\partial T}\right)_V\)
    \(pdg_{6682} = \frac{d}{d pdg_{7343}} pdg_{5786}\)
no validation is available for declarations 1085150613:
1085150613:
12 declare initial expr
  1. 9781951738; locally 9670239:
    \(\kappa_T = \frac{-1}{V} \left( \frac{ \partial V}{\partial P} \right)_T\)
    \(pdg_{4645} = - \frac{\frac{d}{d pdg_{8134}} pdg_{7586}}{pdg_{7586}}\)
no validation is available for declarations 9781951738: error for dim with 9781951738
 9781951738: N/A
Physics Derivation Graph: Steps for first law of thermodynamics

Symbols for this derivation

See also all 227 symbols
symbol ID category latex scope dimension name value Used in derivations references
7586 variable V
\(V\)		 real
  • length: 3
 volume 15
3434 variable \Omega
\(\Omega\)		 real dimensionless number of microscopic configurations (known as microstates) that are consistent with the macroscopic quantities that characterize the system 1
5480 variable \pi_T
\(\pi_T\)		 real
  • length: 1
  • mass: 1
  • time: -2
 internal pressure at constant temperature 2
1088 variable W
\(W\)		 real
  • length: 2
  • mass: 1
  • time: -2
 work done to a system 1
8134 variable P
\(P\)		 real
  • length: -1
  • mass: 1
  • time: -2
 pressure 13
1157 constant k_{Boltzmann}
\(k_{Boltzmann}\)		 ['real']
  • length: 2
  • mass: 1
  • temperature: -1
  • time: -2
 Boltzmann constant 1.38064852 10^{-23}   meter^2 kilogram second^-2 Kelvin^-1
 1
9432 variable Q
\(Q\)		 real
  • length: 2
  • mass: 1
  • time: -2
 heat flow 1
4645 variable \kappa_T
\(\kappa_T\)		 real dimensionless coefficient of isothermal compressibility
  • str_note
6
7343 variable T
\(T\)		 real
  • temperature: 1
 temperature 18
5786 variable U
\(U\)		 real
  • length: 2
  • mass: 1
  • time: -2
 internal energy 7
1394 variable S
\(S\)		 real
  • length: 2
  • mass: 1
  • temperature: -1
  • time: -2
 entropy 1
6682 variable C_V
\(C_V\)		 real
  • length: 2
  • mass: 1
  • temperature: -1
  • time: -2
 heat capacity at constant volume 2
4686 variable \alpha
\(\alpha\)		 real
  • temperature: -1
 expansion coefficient 6
MESSAGES: