This page contains three views of the steps in the derivation: d3js, graphviz PNG, and a table.
Notes for this derivation:
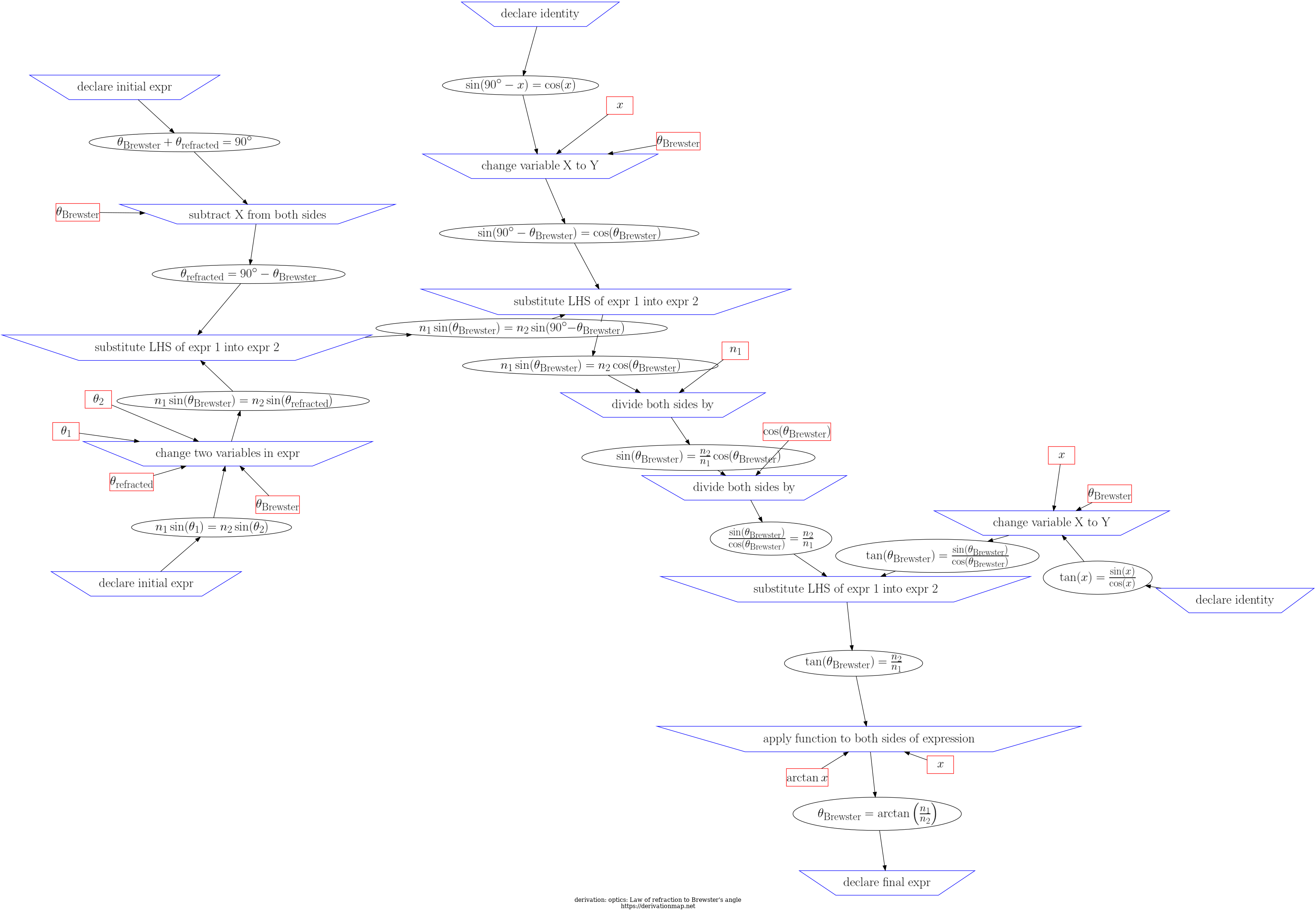
\cite{2001_HRW}; see figure 34-27 on page 824
| Index | Inference Rule | Input latex | Feeds latex | Output latex | step validity | dimension check | unit check | notes |
|---|---|---|---|---|---|---|---|---|
| 6 | declare identity |
|
|
|
no validation is available for declarations |
8588429722:
|
8588429722:
|
|
| 8 | substitute LHS of expr 1 into expr 2 |
|
|
|
Nothing to split |
6831637424:
7696214507: 3061811650: |
6831637424:
7696214507: 3061811650: |
|
| 15 | declare final expr |
|
|
|
no validation is available for declarations |
8495187962:
|
8495187962:
|
|
| 3 | declare initial expr |
|
|
|
no validation is available for declarations |
6450985774:
|
6450985774:
|
|
| 11 | declare identity |
|
|
|
no validation is available for declarations |
4968680693:
|
4968680693:
|
|
| 9 | divide both sides by |
|
|
|
valid |
3061811650:
9756089533: |
3061811650:
9756089533: |
|
| 5 | substitute LHS of expr 1 into expr 2 |
|
|
|
Nothing to split |
1310571337:
no LHS/RHS split 2575937347: 7696214507: |
1310571337:
N/A 2575937347: 7696214507: |
|
| 4 | change two variables in expr |
|
|
|
valid |
6450985774:
2575937347: |
6450985774:
2575937347: |
|
| 12 | change variable X to Y |
|
|
|
LHS diff is tan(pdg1464) - tan(pdg4928) RHS diff is tan(pdg1464) - tan(pdg4928) |
4968680693:
4501377629: |
4968680693:
4501377629: |
|
| 13 | substitute LHS of expr 1 into expr 2 |
|
|
|
valid |
4501377629:
2768857871: 3417126140: |
4501377629:
2768857871: 3417126140: |
|
| 1 | declare initial expr |
|
|
|
no validation is available for declarations |
8945218208:
no LHS/RHS split |
8945218208:
N/A |
|
| 14 | apply function to both sides of expression |
|
|
|
no check performed |
3417126140:
8495187962: |
3417126140:
8495187962: |
|
| 10 | divide both sides by |
|
|
|
valid |
9756089533:
2768857871: |
9756089533:
2768857871: |
|
| 7 | change variable X to Y |
|
|
|
Nothing to split |
8588429722:
6831637424: |
8588429722:
6831637424: |
|
| 2 | subtract X from both sides |
|
|
|
Nothing to split |
8945218208:
no LHS/RHS split 1310571337: no LHS/RHS split |
8945218208:
N/A 1310571337: N/A |
| symbol ID | category | latex | scope | dimension | name | value | Used in derivations | references | |
|---|---|---|---|---|---|---|---|---|---|
| 1958 | variable |
n_2
\(n_2\) |
real | dimensionless | index of refraction for material 2 | 8 | |||
| 7545 | variable |
\theta_2
\(\theta_2\) |
real | dimensionless | angle | 2 | |||
| 2941 | variable |
n_1
\(n_1\) |
['real'] | dimensionless | index of refraction for material 1 | 9 | |||
| 1464 | variable |
x
\(x\) |
['real'] | dimensionless |
|
140 | |||
| 3509 | variable |
\theta_1
\(\theta_1\) |
real | dimensionless | angle | 2 | |||
| 4928 | variable |
\theta_{\rm Brewster}
\(\theta_{\rm Brewster}\) |
['real'] | dimensionless | Brewster's angle | 16 | |||
| 2243 | variable |
\theta_{\rm refracted}
\(\theta_{\rm refracted}\) |
real | dimensionless | refracted angle |
|
2 |