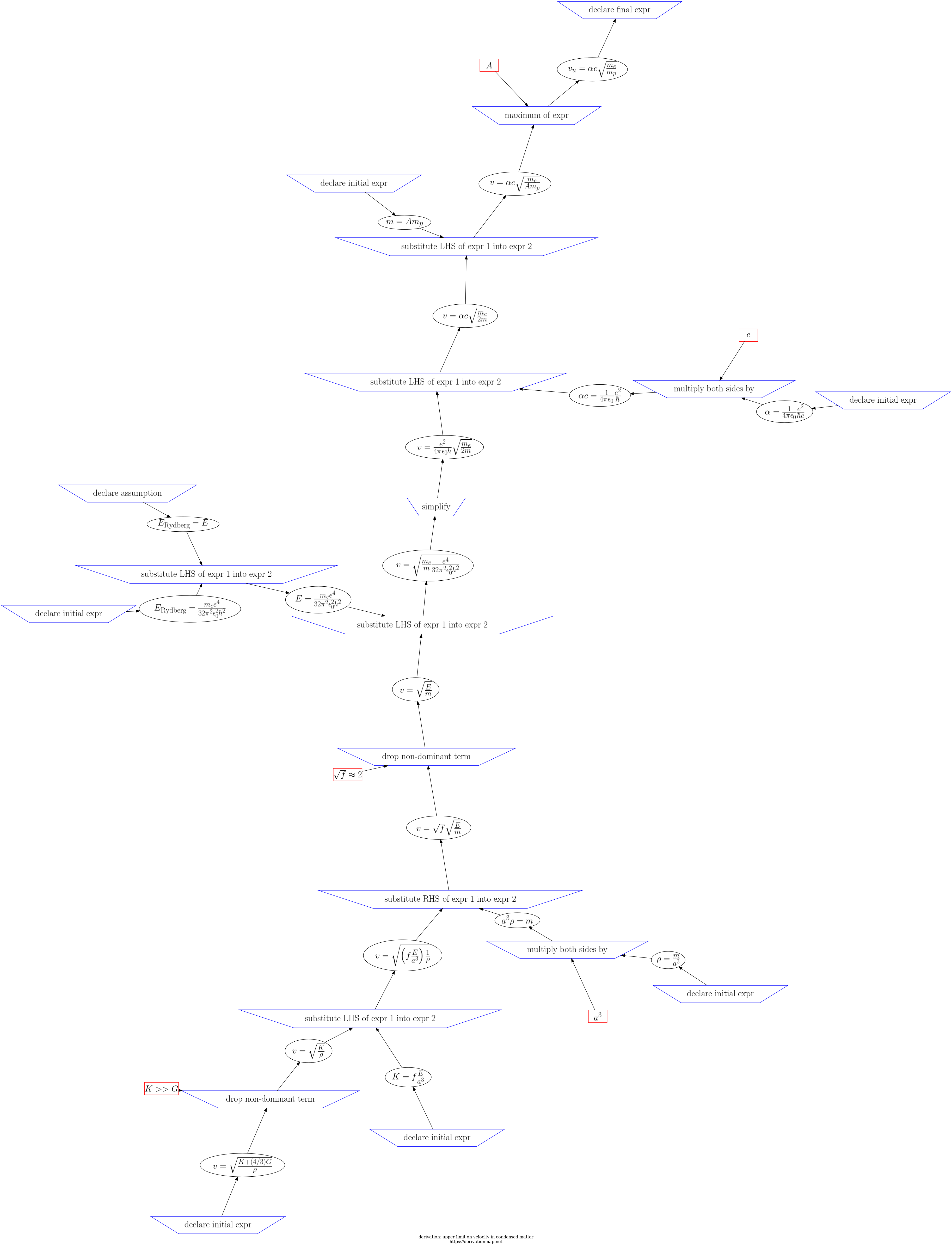
This page contains three views of the steps in the derivation: d3js, graphviz PNG, and a table.
Notes for this derivation:
https://arxiv.org/pdf/2004.04818.pdf
| Index | Inference Rule | Input latex | Feeds latex | Output latex | step validity | dimension check | unit check | notes |
|---|---|---|---|---|---|---|---|---|
| 11 | declare initial expr |
|
|
|
no validation is available for declarations |
8106885760:
|
8106885760:
|
|
| 12 | multiply both sides by |
|
|
|
valid |
8106885760:
5838268428: failed |
8106885760:
5838268428: N/A |
|
| 9 | declare initial expr |
|
|
|
no validation is available for declarations |
1556389363:
|
1556389363:
|
|
| 7 | substitute RHS of expr 1 into expr 2 |
|
|
|
LHS diff is 0 RHS diff is -sqrt(pdg6235)*sqrt(pdg2241/pdg9863) + sqrt(pdg2241*pdg6235/(pdg3935*pdg5854**3)) |
8688588981:
8090924099: 7837519722: |
8688588981:
8090924099: 7837519722: |
|
| 19 | maximum of expr |
|
|
|
no check performed |
2897612567:
7701249282: |
2897612567:
7701249282: |
|
| 18 | substitute LHS of expr 1 into expr 2 |
|
|
|
LHS diff is 0 RHS diff is pdg1370*pdg4567*sqrt(pdg2515/(pdg3285*pdg5916))*(-2 + sqrt(2))/2 |
5646314683:
5789289057: error for dim with 5789289057 2897612567: |
5646314683:
5789289057: N/A 2897612567: |
|
| 20 | declare final expr |
|
|
|
no validation is available for declarations |
7701249282:
|
7701249282:
|
|
| 13 | substitute LHS of expr 1 into expr 2 |
|
|
|
valid |
4107032818:
1556389363: 3291685884: |
4107032818:
1556389363: 3291685884: |
|
| 10 | declare assumption |
|
|
|
no validation is available for declarations |
4107032818:
|
4107032818:
|
|
| 15 | simplify |
|
|
|
LHS diff is 0 RHS diff is sqrt(2)*(pdg1054*pdg3141*pdg7940*sqrt(pdg1999**4*pdg2515/(pdg1054**2*pdg3141**2*pdg7940**2*pdg9863)) - pdg1999**2*sqrt(pdg2515/pdg9863))/(8*pdg1054*pdg3141*pdg7940) |
3935058307:
9640720571: failed |
3935058307:
9640720571: N/A |
|
| 6 | multiply both sides by |
|
|
|
valid |
8908736791:
8688588981: |
8908736791:
8688588981: |
|
| 2 | declare initial expr |
|
|
|
no validation is available for declarations |
9376481176:
|
9376481176:
|
|
| 5 | declare initial expr |
|
|
|
no validation is available for declarations |
8908736791:
|
8908736791:
|
|
| 4 | substitute LHS of expr 1 into expr 2 |
|
|
|
valid |
9376481176:
6504442697: 8090924099: |
9376481176:
6504442697: 8090924099: |
|
| 1 | declare initial expr |
|
|
|
no validation is available for declarations |
4560648264:
|
4560648264:
|
|
| 14 | substitute LHS of expr 1 into expr 2 |
|
|
|
valid |
3291685884:
9854442418: 3935058307: |
3291685884:
9854442418: 3935058307: |
|
| 17 | declare initial expr |
|
|
|
no validation is available for declarations |
5646314683:
|
5646314683:
|
|
| 8 | drop non-dominant term |
|
|
|
no check performed |
7837519722:
9854442418: |
7837519722:
9854442418: |
|
| 3 | drop non-dominant term |
|
|
|
no check performed |
4560648264:
6504442697: |
4560648264:
6504442697: |
|
| 16 | substitute LHS of expr 1 into expr 2 |
|
|
|
LHS diff is 0 RHS diff is sqrt(2)*sqrt(pdg2515/pdg9863)*(-4*pdg1054*pdg1370*pdg3141*pdg4567*pdg7940 + pdg1999**2)/(8*pdg1054*pdg3141*pdg7940) |
5838268428:
failed 9640720571: failed 5789289057: error for dim with 5789289057 |
5838268428:
N/A 9640720571: N/A 5789289057: N/A |
| symbol ID | category | latex | scope | dimension | name | value | Used in derivations | references | |
|---|---|---|---|---|---|---|---|---|---|
| 3285 | variable |
A
\(A\) |
real |
|
atomic mass | 3 | |||
| 4567 | constant |
c
\(c\) |
['real'] |
|
speed of light in vacuum |
299792458 meters/second |
32 | ||
| 6235 | variable |
f
\(f\) |
real | dimensionless | proportionality constant |
|
5 | ||
| 2077 | variable |
v
\(v\) |
real |
|
longitudinal speed of sound in condensed matter | 9 | |||
| 5916 | constant |
m_p
\(m_p\) |
real |
|
mass of proton |
1.67262192369E^{-27} kg |
3 | ||
| 7940 | constant |
\epsilon_0
\(\epsilon_0\) |
real |
|
vacuum permittivity, permittivity of free space or electric constant or the distributed capacitance of the vacuum |
8.8541878128E-{12} F/m |
14 | ||
| 2515 | constant |
m_e
\(m_e\) |
real |
|
mass of electron |
9.1093837015E^{-31} kg |
7 | ||
| 9863 | variable |
m
\(m\) |
real |
|
mass of atom or molecule | 8 | |||
| 3141 | constant |
\pi
\(\pi\) |
['real'] | dimensionless | pi |
3.1415 dimensionless |
|
72 | |
| 4635 | constant |
v_u
\(v_u\) |
real |
|
upper limit on velocity in condensed matter |
36100 m/s |
1 | ||
| 2241 | variable |
E
\(E\) |
real | dimensionless | bonding energy |
|
6 | ||
| 1466 | variable |
K
\(K\) |
real |
|
bulk modulus | 2 | |||
| 5854 | variable |
a
\(a\) |
real |
|
atomic separation |
|
5 | ||
| 1999 | constant |
e
\(e\) |
['real'] |
|
charge of an electron |
1.602*10^{-19} Columb |
6 | ||
| 3935 | variable |
\rho
\(\rho\) |
real |
|
density | 7 | |||
| 9838 | variable |
E_{\rm Rydberg}
\(E_{\rm Rydberg}\) |
real | dimensionless | Rydberg energy | 2 | |||
| 3033 | variable |
G
\(G\) |
real |
|
shear modulus | 2 | |||
| 1370 | constant |
\alpha
\(\alpha\) |
['real'] | dimensionless | fine-structure constant |
1/137.03599999 dimensionless |
5 | ||
| 1054 | constant |
\hbar
\(\hbar\) |
['real'] |
|
Reduced Planck's constant |
1.0545718*10^{-34} meter^2 kilogram second^-1 |
33 |