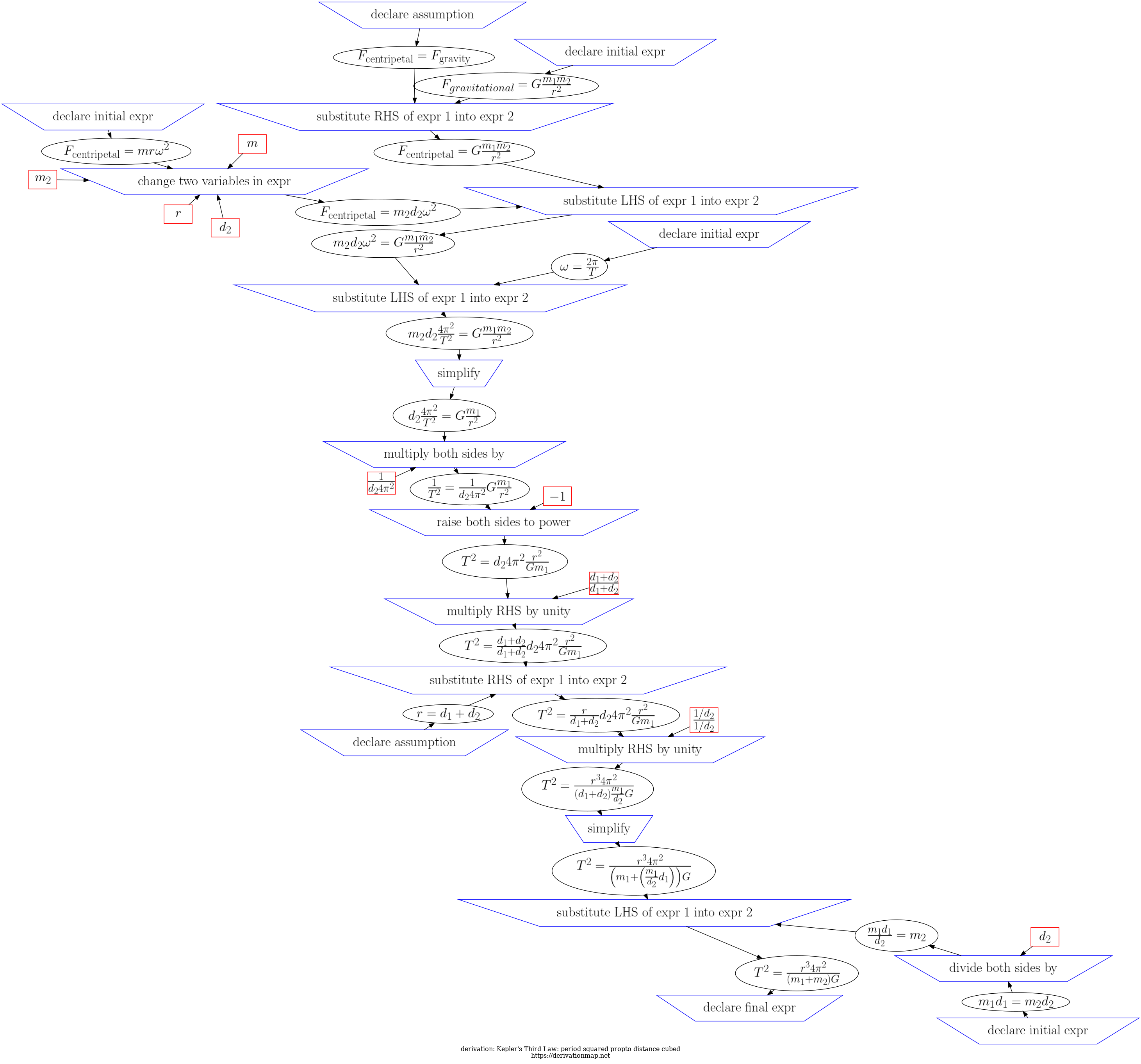
Physics Derivation Graph navigation Sign in

review derivation: Kepler's Third Law: period squared propto distance cubed

This page contains three views of the steps in the derivation: d3js, graphviz PNG, and a table.


Hold the mouse over a node to highlight that node and its neighbors. You can zoom in/out. You can pan the image. You can move nodes by clicking and dragging.

Notes for this derivation:
https://en.wikipedia.org/wiki/Kepler%27s_laws_of_planetary_motion#Third_law

Options
Alternate views of this derivation:
Edit this content:    

To edit a step, click on the number in the "Index" column in the table below

Clicking on the step index will take you to the page where you can edit that step.

Index Inference Rule Input latex Feeds latex Output latex step validity dimension check unit check notes
8 substitute LHS of expr 1 into expr 2
  1. 3132131132; locally 2340248:
    \(\omega = \frac{2\pi}{T}\)
    \(pdg_{2321} = \frac{2 pdg_{3141}}{pdg_{9491}}\)
  2. 3896798826; locally 9388996:
    \(m_2 d_2 \omega^2 = G \frac{m_1 m_2}{r^2}\)
    \(pdg_{2321}^{2} pdg_{2798} pdg_{4851} = \frac{pdg_{4851} pdg_{5022} pdg_{6277}}{pdg_{2530}^{2}}\)
  1. 9070394000; locally 4575586:
    \(m_2 d_2 \frac{4 \pi^2}{T^2} = G \frac{m_1 m_2}{r^2}\)
    \(\frac{4 pdg_{2798} pdg_{3141}^{2} pdg_{4851}}{pdg_{9491}^{2}} = \frac{pdg_{4851} pdg_{5022} pdg_{6277}}{pdg_{2530}^{2}}\)
valid 3132131132:
3896798826:
9070394000:
3132131132:
3896798826:
9070394000:
19 substitute LHS of expr 1 into expr 2
  1. 2217103163; locally 9110206:
    \(\frac{m_1 d_1}{d_2} = m_2\)
    \(\frac{pdg_{5022} pdg_{7652}}{pdg_{2798}} = pdg_{4851}\)
  2. 4188580242; locally 1709969:
    \(T^2 = \frac{r^3 4 \pi^2}{\left(m_1+\left(\frac{m_1}{d_2}d_1\right)\right)G}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{3} pdg_{3141}^{2}}{pdg_{6277} \left(pdg_{5022} + \frac{pdg_{5022} pdg_{7652}}{pdg_{2798}}\right)}\)
  1. 5658865948; locally 8711868:
    \(T^2 = \frac{r^3 4 \pi^2}{(m_1+m_2)G}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{3} pdg_{3141}^{2}}{pdg_{6277} \left(pdg_{4851} + pdg_{5022}\right)}\)
valid 2217103163:
4188580242:
5658865948:
2217103163:
4188580242:
5658865948:
7 declare initial expr
  1. 3132131132; locally 2340248:
    \(\omega = \frac{2\pi}{T}\)
    \(pdg_{2321} = \frac{2 pdg_{3141}}{pdg_{9491}}\)
no validation is available for declarations 3132131132:
3132131132:
12 multiply RHS by unity
  1. 9170048197; locally 7795202:
    \(T^2 = d_2 4 \pi^2 \frac{r^2}{G m_1}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{2} pdg_{2798} pdg_{3141}^{2}}{pdg_{5022} pdg_{6277}}\)
  1. 8122039815:
    \(\frac{d_1+d_2}{d_1+d_2}\)
    \(1\)
  1. 1811867899; locally 6577160:
    \(T^2 = \frac{d_1+d_2}{d_1+d_2} d_2 4 \pi^2 \frac{r^2}{G m_1}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{2} pdg_{2798} pdg_{3141}^{2}}{pdg_{5022} pdg_{6277}}\)
valid 9170048197:
1811867899:
9170048197:
1811867899:
3 change two variables in expr
  1. 4393258808; locally 8072137:
    \(F_{\rm centripetal} = m r \omega^2\)
    \(pdg_{1687} = pdg_{2321}^{2} pdg_{2530} pdg_{5156}\)
  1. 8916428651:
    \(m\)
    \(pdg_{5156}\)
  2. 1635147226:
    \(m_2\)
    \(pdg_{4851}\)
  3. 9884115626:
    \(r\)
    \(pdg_{2530}\)
  4. 1036530438:
    \(d_2\)
    \(pdg_{2798}\)
  1. 3649797559; locally 6652843:
    \(F_{\rm centripetal} = m_2 d_2 \omega^2\)
    \(pdg_{1687} = pdg_{2321}^{2} pdg_{2798} pdg_{4851}\)
valid 4393258808: dimensions are consistent
3649797559:
4393258808: N/A
3649797559:
11 raise both sides to power
  1. 9152823411; locally 7556753:
    \(\frac{1}{T^2} = \frac{1}{d_2 4 \pi^2} G \frac{m_1}{r^2}\)
    \(\frac{1}{pdg_{9491}^{2}} = \frac{pdg_{5022} pdg_{6277}}{4 pdg_{2530}^{2} pdg_{2798} pdg_{3141}^{2}}\)
  1. 7445388869:
    \(-1\)
    \(-1\)
  1. 9170048197; locally 7795202:
    \(T^2 = d_2 4 \pi^2 \frac{r^2}{G m_1}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{2} pdg_{2798} pdg_{3141}^{2}}{pdg_{5022} pdg_{6277}}\)
no check is performed 9152823411:
9170048197:
9152823411:
9170048197:
6 substitute LHS of expr 1 into expr 2
  1. 3649797559; locally 6652843:
    \(F_{\rm centripetal} = m_2 d_2 \omega^2\)
    \(pdg_{1687} = pdg_{2321}^{2} pdg_{2798} pdg_{4851}\)
  2. 6829281943; locally 4382594:
    \(F_{\rm centripetal} = G \frac{m_1 m_2}{r^2}\)
    \(pdg_{1687} = \frac{pdg_{4851} pdg_{5022} pdg_{6277}}{pdg_{2530}^{2}}\)
  1. 3896798826; locally 9388996:
    \(m_2 d_2 \omega^2 = G \frac{m_1 m_2}{r^2}\)
    \(pdg_{2321}^{2} pdg_{2798} pdg_{4851} = \frac{pdg_{4851} pdg_{5022} pdg_{6277}}{pdg_{2530}^{2}}\)
valid 3649797559:
6829281943:
3896798826:
3649797559:
6829281943:
3896798826:
17 declare initial expr
  1. 5128670694; locally 4476518:
    \(m_1 d_1 = m_2 d_2\)
    \(pdg_{5022} pdg_{7652} = pdg_{2798} pdg_{4851}\)
no validation is available for declarations 5128670694:
5128670694:
16 simplify
  1. 3781109867; locally 6644719:
    \(T^2 = \frac{r^3 4 \pi^2}{(d_1+d_2) \frac{m_1}{d_2}G}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{3} pdg_{2798} pdg_{3141}^{2}}{pdg_{5022} pdg_{6277} \left(pdg_{2798} + pdg_{7652}\right)}\)
  1. 4188580242; locally 1709969:
    \(T^2 = \frac{r^3 4 \pi^2}{\left(m_1+\left(\frac{m_1}{d_2}d_1\right)\right)G}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{3} pdg_{3141}^{2}}{pdg_{6277} \left(pdg_{5022} + \frac{pdg_{5022} pdg_{7652}}{pdg_{2798}}\right)}\)
valid 3781109867:
4188580242:
3781109867:
4188580242:
1 declare initial expr
  1. 1292735067; locally 5331094:
    \(F_{gravitational} = G \frac{m_1 m_2}{r^2}\)
    \(pdg_{2867} = \frac{pdg_{4851} pdg_{5022} pdg_{6277}}{pdg_{2530}^{2}}\)
no validation is available for declarations 1292735067:
1292735067:
10 multiply both sides by
  1. 9838128064; locally 6210646:
    \(d_2 \frac{4 \pi^2}{T^2} = G \frac{m_1}{r^2}\)
    \(\frac{4 pdg_{2798} pdg_{3141}^{2}}{pdg_{9491}^{2}} = \frac{pdg_{5022} pdg_{6277}}{pdg_{2530}^{2}}\)
  1. 5684907106:
    \(\frac{1}{d_2 4 \pi^2}\)
    \(\frac{1}{4 pdg_{2798} pdg_{3141}^{2}}\)
  1. 9152823411; locally 7556753:
    \(\frac{1}{T^2} = \frac{1}{d_2 4 \pi^2} G \frac{m_1}{r^2}\)
    \(\frac{1}{pdg_{9491}^{2}} = \frac{pdg_{5022} pdg_{6277}}{4 pdg_{2530}^{2} pdg_{2798} pdg_{3141}^{2}}\)
valid 9838128064:
9152823411:
9838128064:
9152823411:
20 declare final expr
  1. 5658865948; locally 8711868:
    \(T^2 = \frac{r^3 4 \pi^2}{(m_1+m_2)G}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{3} pdg_{3141}^{2}}{pdg_{6277} \left(pdg_{4851} + pdg_{5022}\right)}\)
no validation is available for declarations 5658865948:
5658865948:
period squared propto distance cubed
15 multiply RHS by unity
  1. 2906548078; locally 8324356:
    \(T^2 = \frac{r}{d_1+d_2} d_2 4 \pi^2 \frac{r^2}{G m_1}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{3} pdg_{2798} pdg_{3141}^{2}}{pdg_{5022} pdg_{6277} \left(pdg_{2798} + pdg_{7652}\right)}\)
  1. 9524810853:
    \(\frac{1/d_2}{1/d_2}\)
    \(1\)
  1. 3781109867; locally 6644719:
    \(T^2 = \frac{r^3 4 \pi^2}{(d_1+d_2) \frac{m_1}{d_2}G}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{3} pdg_{2798} pdg_{3141}^{2}}{pdg_{5022} pdg_{6277} \left(pdg_{2798} + pdg_{7652}\right)}\)
valid 2906548078:
3781109867:
2906548078:
3781109867:
13 declare assumption
  1. 5586102077; locally 8233899:
    \(r = d_1 + d_2\)
    \(pdg_{2530} = pdg_{2798} + pdg_{7652}\)
no validation is available for declarations 5586102077:
5586102077:
5 substitute RHS of expr 1 into expr 2
  1. 3176662571; locally 2600680:
    \(F_{\rm centripetal} = F_{\rm gravity}\)
    \(pdg_{2867} = pdg_{1687}\)
  2. 1292735067; locally 5331094:
    \(F_{gravitational} = G \frac{m_1 m_2}{r^2}\)
    \(pdg_{2867} = \frac{pdg_{4851} pdg_{5022} pdg_{6277}}{pdg_{2530}^{2}}\)
  1. 6829281943; locally 4382594:
    \(F_{\rm centripetal} = G \frac{m_1 m_2}{r^2}\)
    \(pdg_{1687} = \frac{pdg_{4851} pdg_{5022} pdg_{6277}}{pdg_{2530}^{2}}\)
LHS diff is -pdg1687 + pdg2867 RHS diff is 0 3176662571:
1292735067:
6829281943:
3176662571:
1292735067:
6829281943:
18 divide both sides by
  1. 5128670694; locally 4476518:
    \(m_1 d_1 = m_2 d_2\)
    \(pdg_{5022} pdg_{7652} = pdg_{2798} pdg_{4851}\)
  1. 8044416349:
    \(d_2\)
    \(pdg_{2798}\)
  1. 2217103163; locally 9110206:
    \(\frac{m_1 d_1}{d_2} = m_2\)
    \(\frac{pdg_{5022} pdg_{7652}}{pdg_{2798}} = pdg_{4851}\)
valid 5128670694:
2217103163:
5128670694:
2217103163:
4 declare assumption
  1. 3176662571; locally 2600680:
    \(F_{\rm centripetal} = F_{\rm gravity}\)
    \(pdg_{2867} = pdg_{1687}\)
no validation is available for declarations 3176662571:
3176662571:
9 simplify
  1. 9070394000; locally 4575586:
    \(m_2 d_2 \frac{4 \pi^2}{T^2} = G \frac{m_1 m_2}{r^2}\)
    \(\frac{4 pdg_{2798} pdg_{3141}^{2} pdg_{4851}}{pdg_{9491}^{2}} = \frac{pdg_{4851} pdg_{5022} pdg_{6277}}{pdg_{2530}^{2}}\)
  1. 9838128064; locally 6210646:
    \(d_2 \frac{4 \pi^2}{T^2} = G \frac{m_1}{r^2}\)
    \(\frac{4 pdg_{2798} pdg_{3141}^{2}}{pdg_{9491}^{2}} = \frac{pdg_{5022} pdg_{6277}}{pdg_{2530}^{2}}\)
LHS diff is 4*pdg2798*pdg3141**2*(pdg4851 - 1)/pdg9491**2 RHS diff is pdg5022*pdg6277*(pdg4851 - 1)/pdg2530**2 9070394000:
9838128064:
9070394000:
9838128064:
14 substitute RHS of expr 1 into expr 2
  1. 5586102077; locally 8233899:
    \(r = d_1 + d_2\)
    \(pdg_{2530} = pdg_{2798} + pdg_{7652}\)
  2. 1811867899; locally 6577160:
    \(T^2 = \frac{d_1+d_2}{d_1+d_2} d_2 4 \pi^2 \frac{r^2}{G m_1}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{2} pdg_{2798} pdg_{3141}^{2}}{pdg_{5022} pdg_{6277}}\)
  1. 2906548078; locally 8324356:
    \(T^2 = \frac{r}{d_1+d_2} d_2 4 \pi^2 \frac{r^2}{G m_1}\)
    \(pdg_{9491}^{2} = \frac{4 pdg_{2530}^{3} pdg_{2798} pdg_{3141}^{2}}{pdg_{5022} pdg_{6277} \left(pdg_{2798} + pdg_{7652}\right)}\)
LHS diff is 0 RHS diff is 4*pdg2530**2*pdg2798*pdg3141**2*(-pdg2530 + pdg2798 + pdg7652)/(pdg5022*pdg6277*(pdg2798 + pdg7652)) 5586102077:
1811867899:
2906548078:
5586102077:
1811867899:
2906548078:
2 declare initial expr
  1. 4393258808; locally 8072137:
    \(F_{\rm centripetal} = m r \omega^2\)
    \(pdg_{1687} = pdg_{2321}^{2} pdg_{2530} pdg_{5156}\)
no validation is available for declarations 4393258808: dimensions are consistent
 4393258808: N/A
Physics Derivation Graph: Steps for Kepler's Third Law: period squared propto distance cubed

Symbols for this derivation

See also all 227 symbols
symbol ID category latex scope dimension name value Used in derivations references
6277 constant G
\(G\)		 real
  • length: 3
  • mass: -1
  • time: -2
 gravitational constant 6.67430*10^{-11}   m^3 * kg^-1 * s^-2
 60
2798 variable d_2
\(d_2\)		 real
  • length: 1
 distance 18
2530 variable r
\(r\)		 ['real']
  • length: 1
 radius 60
2867 variable F_{\rm gravity}
\(F_{\rm gravity}\)		 real
  • length: 1
  • mass: 1
  • time: -2
 force due to gravity 12
5022 variable m_1
\(m_1\)		 real
  • mass: 1
 mass 35
4851 variable m_2
\(m_2\)		 real
  • mass: 1
 mass 31
3141 constant \pi
\(\pi\)		 ['real'] dimensionless pi 3.1415   dimensionless
 72
5156 variable m
\(m\)		 ['real']
  • mass: 1
 mass 69
1687 variable F_{\rm centripetal}
\(F_{\rm centripetal}\)		 real
  • length: 1
  • mass: 1
  • time: -2
 centripetal force 8
2321 variable \omega
\(\omega\)		 ['real']
  • time: -1
 angular frequency 26
7652 variable d_1
\(d_1\)		 real
  • length: 1
 distance 8
9491 variable T
\(T\)		 ['real']
  • time: 1
 period 20
MESSAGE: